Prismatic Cohomology: An Overview

May 8, 2023May 15, 2023 / Anton Hilado / 1 Comment

In p-adic Hodge Theory: An Overview, we discussed how the different cohomologies of some smooth projective variety over the p-adic numbers (or some finite extension of it) such as its p-adic etale cohomology, de Rham cohomology, or crystalline cohomology can be related to each other via the machinery of period rings.

We note that the theories we have discussed in p-adic Hodge Theory: An Overview are “rational”, in that they involve cohomologies with “rational” coefficients (for instance $H_{\mathrm{et}}^{1}(X,\mathbb{Q}_{\ell})$ ). Cohomology with integral coefficients holds some information that gets lost when passing to rational coefficients; for instance, all the information about the torsion is lost. If we want to relate, say, torsion subgroups of different cohomologies to each other, we would need some sort of integral p-adic Hodge theory. In this post, we will discuss one approach to integral p-adic Hodge theory, called prismatic cohomology, developed by Bhargav Bhatt and Peter Scholze.

As a preliminary to defining prismatic cohomology, we briefly discuss the concept of $\delta$ -rings. A $\delta$ -ring is a ring $R$ together with a map $\delta:R\to R$ called a p-derivation, satisfying the following properties:

$\displaystyle \delta(xy)=\delta(x)+\delta(y)+\frac{x^{p}+y^{p}-(x+y)^{p}}{p}$

$\displaystyle \delta(xy)=x^{p}\delta(y)+y^{p}\delta(x)+p\delta(y)\delta(x)$

$\displaystyle \delta(1)=\delta(0)=0$

The concept of $\delta$ -rings were introduced by Andre Joyal, and its theory and that of p-derivations was further developed by Alexandru Buium. This also has connections to the work of James Borger as we discussed in The Field with One Element.

A prism is a pair $(A,I)$ consisting of a $\delta$ -ring $A$ an ideal $I$ defining a Cartier divisor on $\mathrm{Spec}(A)$ , such that $A$ is derived $(p,I)$ -complete (this means that for every $f\in(p,I)$ we have, considering $A$ as a module over itself, $\mathrm{Hom}(A_{f},A)=0$ and $\mathrm{Ext}_{A}^{1}(A_{f},A)=0$ ) and $p\in I+\phi(I)A$ .

An example of a prism is given by taking $A=\mathbb{Z}_{p}[[u]]$ and taking $I=(u-p)$ . Another important example that we will show up again later in this post is given by $A=A_{\mathrm{inf}}(\mathcal{O}_{\mathbb{C}_{p}})$ and $I=\mathrm{ker}(\theta)$ , where $\theta:A_{\mathrm{inf}}(\mathcal{O}_{\mathbb{C}_{p}})\to \mathcal{O}_{\mathbb{C}_{p}}$ is the canonical map (see also Perfectoid Fields for a discussion of these concepts).

Prisms are also related to (integral) perfectoid rings (see also Adic Spaces and Perfectoid Spaces). A prism $(A,I)$ is perfect if the ring $A$ is perfect (i.e. the Frobenius is an automorphism). If $(A,I)$ is a perfect prism, then the quotient $A/I$ is an perfectoid ring. In fact, there is an equivalence of categories between perfect prisms and perfectoid rings, and one can go the other way via the $A_{\mathrm{inf}}$ construction, i.e. given a perfectoid ring $R$ we set $A=W(R^{\flat})$ , and there is a canonical map $\theta:W(R^{\flat})\to R$ , and we set $I=\mathrm{ker}({\theta})$ ; then the pair $(A,I)$ obtained via this construction is a prism.

Remark: The perfectoid rings mentioned in the previous paragraph are related, but not quite the same, as in Adic Spaces and Perfectoid Spaces. The perfectoid rings in the previous paragraph and in the rest of the article correspond to the ring of integral elements of the perfectoid rings in Adic Spaces and Perfectoid Spaces, hence the word “integral”. To match with the references, we will drop the word “integral” in the rest of this post, but perfectoid rings in this post will mean integral perfectoid rings.

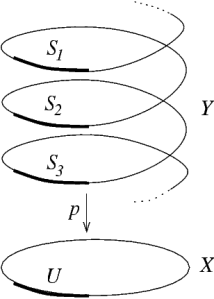
Let $(A,I)$ be a prism and let $R$ be a formally smooth $A/I$ -algebra. The prismatic site of $R$ relative to $A$ , denoted $(R/A)_{\Delta}$ , is the category of prisms $(B, IB)$ over $A$ together with a map from $R$ to $B/IB$ over $A$ . We write such an object as $(R\rightarrow B/IB\leftarrow B)$ . We have functors $\mathcal{O}_{\Delta}$ and $\overline{\mathcal{O}}_{\Delta}$ which send $(R\rightarrow B/IB\leftarrow B)$ to $B$ and $B/IB$ respectively. We now define the prismatic cohomology of $R$ , denoted $\Delta_{R/A}$ , to be $R\Gamma((R/A)_{\Delta},\mathcal{O})$ . As an example, in the special case that $R=A/I$ , then the prismatic cohomology $\Delta_{R/A}$ is just $A$ .

Related to prisms and prismatic cohomology is the notion of prismatic crystals. A prismatic crystal is an assignment, given a prism $(B,J)$ in $\Delta_{R/A}$ , of a finite projective $B$ -module. We also have related notions of Hodge-Tate crystals (which assign finite projective $B/J$ -modules instead), prismatic F-crystals (prismatic crystals $\mathcal{E}$ with an isomorphism $\phi^{*}\mathcal{E}[1/I]\to\mathcal{E}[1/I]$ ), and notions of crystals where instead of modules we consider complexes (perfect or $(p,I)$ -complete) of modules.

The prismatic cohomology $\Delta_{R/A}$ is equipped with some other extra structures, for instance we have the notion of a Breuil-Kisin twist $\Delta_{R/A}\{ 1\}$ , which corresponds to the Tate twist in etale cohomology. The prismatic cohomology also comes with a filtration, called the Nygaard filtration. These structures are important for some of the applications of prismatic cohomology. For instance, the two notions just discussed allows us to give a definition of syntomic cohomology as follows:

$\displaystyle \mathbb{Z}_{p}(i)(R)=\mathrm{fib}(\mathrm{Fil}^{i}\Delta_{R/A}\{i\}\xrightarrow{\phi_{i}-1}\Delta_{R/A}\{i\})$

The syntomic cohomology is related to etale K-theory of p-adically complete rings; in particular, there is a motivic filtration on the etale K-theory whose graded pieces are given by shifts of the syntomic cohomology. This relation between etale K-theory and syntomic cohomology goes through the theory of topological cyclic homology (and other related theories such as topological Hochschild homology) and hints at deep connections between prismatic cohomology and algebraic topology; we leave further discussion of these directions to the references or possible future posts.

As mentioned earlier, prismatic cohomology gives us an integral version of p-adic Hodge theory. Therefore, it must be related to the different cohomology theories such as crystalline cohomology and etale cohomology. This relationship is explicitly stated in the form of the following comparison theorems. For crystalline cohomology, we have (in the language of derived categories)

$\displaystyle R\Gamma_{\mathrm{crys}}(R/A)\cong \phi_{A}^{*}\Delta_{R/A}$

The crystalline cohomology can be considered a “lift” of the de Rham cohomology; namely the de Rham complex $\Omega_{R/(A/p)}^{\bullet}$ that computes the de Rham cohomology of $R$ over $A/(p)$ is related to $R\Gamma_{\mathrm{crys}}(R/A)$ as follows:

$\displaystyle R\Gamma_{\mathrm{crys}}(R/A)\otimes_{A}^{\mathbb{L}}A/p=\Omega_{R/(A/p)}^{\bullet}$

And so we see that prismatic cohomology also computes de Rham cohomology. Meanwhile, for etale cohomology, letting $d$ be an element of $A$ such that $\delta(d)\in A^{\times}$ (we call such an element $d$ a distinguished element), we have

$\displaystyle R\Gamma_{\mathrm{et}}(\mathrm{Spec}(R)[1/p],\mathbb{Z}/p^{n})\cong (\Delta_{R/A}[1/d]/p^{n})^{\phi=1}$

These comparison theorems of prismatic cohomology can be used to obtain results regarding cohomology with torsion coefficients. For instance, let $X$ be a proper formal scheme over $\mathcal{O}_{\mathbb{C}_{p}}$ . Let $(A,I)$ be the prism given by $A=A_{\mathrm{inf}}(\mathcal{O}_{\mathbb{C}_{p}})$ and $I=\mathrm{ker}(\theta)$ . Then one can construct the prismatic cohomology $\Delta_{X/A}$ by gluing together the different $\Delta_{R/A}$ for every $\mathrm{Spf}(R)\subset X$ , and then use the comparison theorems previously mentioned to prove the following result:

$\displaystyle \mathrm{dim}_{\mathbb{F}_{p}}H^{i}(X^{\mathrm{an}},\mathbb{Z}/p)\leq\mathrm{dim}_{\mathbb{F}_{p}}H_{\mathrm{dR}}^{i}(X)$

Note that this result involves torsion coefficients, and therefore inaccessible if one does not have an “integral” p-adic Hodge theory.

Another application of ideas from prismatic cohomology is the construction of a p-adic Riemann-Hilbert correspondence by Bhargav Bhatt and Jacob Lurie. The classical Riemann-Hilbert correspondence is an equivalence of derived categories between regular holonomic D-modules and Zariski constructible sheaves, itself an upgrade of a classical equivalence of categories between algebraic vector bundles with regular flat connection (which we may think of as differential equations) and local systems of complex vector spaces (which we may think of as solutions to these differential equations) on a smooth complex algebraic variety.

Bhatt and Lurie have developed a version of the Riemann-Hilbert correspondence for perfectoid spaces, making use of perfected prismatic cohomology, and the way it allows us to construct a “perfectoidization” of a ring. Given any ring, its perfectoidization is an $R$ -algebra $R_{\mathrm{pfd}}$ which is perfectoid, and such that for any other perfectoid ring $S$ , $\mathrm{Hom}(R_{\mathrm{pfd}},S)\cong \mathrm{Hom}(R,S)$ . As we just stated, a way to construct the perfectoidization is given by the perfected prismatic cohomology. Let $R=A/I$ be perfectoid. We define the following complex:

$\displaystyle \Delta_{R/A,\mathrm{perf}}=\varinjlim(\Delta_{R/A}\xrightarrow{\phi}\Delta_{R/A}\xrightarrow{\phi}\ldots)^{\wedge}$

Now let $S$ be a finite $R$ -algebra, and $Y=\mathrm{Spec}(S)$ . The pair $(H_{\Delta,\mathrm{perf}}^{0}(Y),IH_{\Delta,\mathrm{perf}}^{0}(Y))$ is a perfect prism, and the quotient $H_{\Delta,\mathrm{perf}}^{0}(Y)/I$ is a perfectoidization of $S$ (which we may therefore also denote by $S_{\mathrm{pfd}}$ ).

Let $f:U\to X$ be an etale morphism. We may express this as the composition $U\xrightarrow{j}\overline{U}\xrightarrow{\overline{f}}X$ , where $\overline{f}$ is finite. We may express $\overline{U}=\mathrm{Spec}(S)$ and $\overline{U}=\mathrm{S/J}$ . Then we can define

$\displaystyle \overline{\Gamma}_{c}(U)=\mathrm{ker}(S_{\mathrm{pfd}}\twoheadrightarrow (S/J)_{\mathrm{pfd}})$

If $R$ is a perfect $\mathbb{F}_{p}$ -algebra, then the functor which sends the sheaf $f_{!}\underline{\mathbb{F}_{p}}$ to $\overline{\Gamma}_{c}(U)$ may be taken to be a Riemann-Hilbert functor, agreeing with previous constructions of Bhatt and Lurie. Inspired by this, in the more general case we can define

$\displaystyle \Gamma_{c}(U)=\mathrm{ker}(H_{\Delta,\mathrm{perf}}^{0}(\overline{U})\twoheadrightarrow H_{\Delta,\mathrm{perf}}^{0}(\partial\overline{U}))$

Note that $\Gamma_{c}(U)$ is an $A$ -module, and that $\overline{\Gamma}_{c}(U)=R\otimes_{A}\Gamma_{c}(U)$ . For an etale morphism $f:U\to X$ , let $h_{U}$ be the sheaf $f_{!}\underline{\mathbb{Z}}$ on $X_{\mathrm{et}}$ , where $\underline{\mathbb{Z}}$ is the constant sheaf of $\mathbb{Z}$ -coefficients. Sheaves of this form generate the category of abelian sheaves on $X_{\mathrm{et}}$ . The Riemann-Hilbert functor for perfectoid spaces is then the functor that takes $h_{U}$ to $\Gamma_{c}(U)$ . Bhatt and Lurie have also extended their Riemann-Hilbert functor not only to perfectoid spaces but also to p-adic formal schemes over $\mathcal{O}_{\mathbb{C}_{p}}$ .

Bhargav Bhatt has used the p-adic Riemann-Hilbert correspondence to prove new results in commutative algebra and algebraic geometry. For instance, Bhatt has used it (in conjunction with other ideas from prismatic cohomology) to prove the following theorem. Suppose $R=\mathbb{Z}[x_{1},\ldots,x_{n}]$ . Let $R^{+}$ denote the integral closure of $R$ inside the algebraic closure of its fraction field. Then, for any prime $p$ , the ring $R^{+}$ is p-adically Cohen-Macaulay, which explicitly means that for any $i$ , $x_{i}$ is a zero divisor of $R^{+}/(p,x_{1},\ldots,x_{i-1}).$ A “global” version of this theorem is mixed-characteristic Kodaira vanishing up to finite covers, also proved by Bhatt, and which has applications to the minimal model program in birational geometry.

Remark: Bhatt and Lurie have also developed another version of the p-adic Riemann-Hilbert correspondence for varieties over $\mathbb{Q}_{p}$ . The methods appear to differ from what has been discussed in this post, instead using a version of the methods developed by Peter Scholze in his work on p-adic Hodge theory for rigid analytic varieties (also very briefly mentioned in The Geometrization of the Local Langlands Correspondence); this version of the p-adic Riemann-Hilbert correspondence has also found applications in algebraic geometry, such as Kollar vanishing.

There is also a “geometrized” version of prismatic cohomology, also developed by Bhatt and Lurie where the prismatic cohomology is obtained from a complex of sheaves on a certain stack called the “Cartier-Witt stack“. The Cartier-Witt stack itself can be generalized (or “relativized”) via the concept of a prismatization $X^{\Delta}$ of a p-adic formal scheme $X$ (the Cartier-Witt stack is the prismatization $\mathrm{Spf}(\mathbb{Z}_{p})^{\Delta}$ of $\mathrm{Spf}(\mathbb{Z}_{p})$ ). The prismatization $X^{\Delta}$ fits into a bigger stack $X^{\mathcal{N}}$ , the Nygaard filtered prismatization of $X$ , together with another copy of $X^{\Delta}$ , and gluing together these two copies results in another stack $X^{\mathrm{Syn}}$ called the syntomification of $X$ .

The derived category of quasi-coherent sheaves on $X^{\mathrm{Syn}}$ is called the category of prismatic F-gauges on $X$ . The theory of prismatic F-gauges is related to the theory of Galois representations. For instance, the etale realization of a coherent sheaf on $\mathbb{Z}_{p}^{\mathrm{Syn}}$ , upon inverting $p$ , is a crystalline Galois representation. The theory also allows us to refine Tate duality for Galois representations. Together, these facts can be used to show that the image of such a coherent sheaf in Galois cohomology is the Bloch-Kato Selmer group, which is a very important object in the theory of L-functions and modularity.

Prismatic cohomology has shown itself to have very many interesting aspects which are currently the subject of much ongoing research. We have only given a very shallow overview of the theory in this post. We leave a list of references for the interested reader for further reading, but also hope to discuss more details of this rapidly growing theory in more depth in future blog posts.

References:

Prisms and Prismatic Cohomology by Bhargav Bhatt and Peter Scholze

Geometric Aspects of p-adic Hodge Theory (notes by Chao Li from a course by Bhargav Bhatt)

Prismatic Cohomology by Bhargav Bhatt

Notes on Prismatic Cohomology by Kiran Kedlaya

Algebraic Geometry in Mixed Characteristic by Bhargav Bhatt

A Riemann-Hilbert Correspondence in p-adic Geometry by Jacob Lurie (2022 Felix Klein Lectures)

Prismatic F-Gauges by Bhargav Bhatt

Absolute Prismatic Cohomology by Bhargav Bhatt and Jacob Lurie

The Prismatization of p-adic Formal Schemes by Bhargav Bhatt and Jacob Lurie

Prismatic / THH Reading List by Yuri Sulyma

Some Useful Links: Knots in Physics and Number Theory

August 28, 2017 / Anton Hilado / 1 Comment

In modern times, “knots” have been important objects of study in mathematics. These “knots” are akin to the ones we encounter in ordinary life, except that they don’t have loose ends. For a better idea of what I mean, consider the following picture of what is known as a “trefoil knot“:

More technically, a knot is defined as the embedding of a circle in 3-dimensional space. For more details on the theory of knots, the reader is referred to the following Wikipedia pages:

Knot on Wikipedia

Knot Theory on Wikipedia

One of the reasons why knots have become such a major part of modern mathematical research is because of the work of mathematical physicists such as Edward Witten, who has related them to the Feynman path integral in quantum mechanics (see Lagrangians and Hamiltonians).

Witten, who is very famous for his work on string theory (see An Intuitive Introduction to String Theory and (Homological) Mirror Symmetry) and for being the first, and so far only, physicist to win the prestigious Fields medal, himself explains the relationship between knot theory and quantum mechanics in the following article:

Knots and Quantum Theory by Edward Witten

But knots have also appeared in other branches of mathematics. For example, in number theory, the result in etale cohomology known as Artin-Verdier duality states that the integers are similar to a 3-dimensional object in some sense. In particular, because it has a trivial etale fundamental group (which is kind of an algebraic analogue of the fundamental group discussed in Homotopy Theory and Covering Spaces), it is similar to a 3-sphere (recall the common but somewhat confusing notation that the ordinary sphere we encounter in everyday life is called the 2-sphere, while a circle is also called the 1-sphere).

Note: The fact that a closed 3-dimensional space with a trivial fundamental group is a 3-sphere is the content of a very famous conjecture known as the Poincare conjecture, proved by Grigori Perelman in the early 2000’s. Perelman refused the million-dollar prize that was supposed to be his reward, as well as the Fields medal.

The prime numbers, because their associated finite fields have one cover for every integer, are like circles, and recalling the definition of knots mentioned above, are therefore like knots on this 3-sphere. This analogy, originally developed by David Mumford and Barry Mazur, is better explained in the following post by Lieven le Bruyn on his blog neverendingbooks:

What is the Knot Associated to a Prime on neverendingbooks

Finally, given what we have discussed, could it be that knot theory can “tie together” (pun intended) physics and number theory? This is the motivation behind the new subject called “arithmetic Chern-Simons theory” which is introduced in the following paper by Minhyong Kim:

Arithmetic Chern-Simons Theory I by Minhyong Kim

Of course, it must also be clarified that this is not the only way by which physics and number theory are related. It is merely another way, a new and not yet thoroughly explored one, by which the unity of mathematics manifests itself via its many different branches helping one another.

Covering Spaces

April 14, 2017 / Anton Hilado / 2 Comments

In Homotopy Theory we defined the fundamental group of a topological space as the group of equivalence classes of “loops” on the space. In this post, we discuss the fundamental group from another point of view, this time making use of the concept of covering spaces. In doing so, we will uncover some interesting analogies with the theory of Galois groups (see Galois Groups). Galois groups are usually associated with number theory, and not usually thought of as being related to algebraic topology, therefore one might find these analogies to be quite surprising and unexpected.

We will start with an example, which we are already somewhat familiar with, the circle. For simplicity, we set the circle to have a circumference equal to $1$ . We also consider the real line, which we will think of as being “wrapped” over the circle, like a spring. We may think of this “spring” as casting a “shadow”, which is the circle. See also the following image by user Yonatan of Wikipedia:

Covering_map

Looking at the diagram, we see that we can map the line to the circle by a kind of “projection”. As we move around the line, we “project” to different points on the circle. However, if we move by any distance equal to an integer multiple of the circumference of the circle (which as we said above we have set equal to $1$ ), we come back to the same point if we project to the circle. At this point we recall that the fundamental group of the circle is the group of integers under addition. We can think of an element of this group (an ordinary integer) as giving the “winding number” of a loop on the circle.

In this example, we refer to the line as a covering space of the circle. Since the line is simply connected (see Homotopy Theory), it is also the universal covering space of the circle. The mapping of one point to another point on the line, such that they both “project” to the same point on the circle, is called a deck transformation. The deck transformations of a covering space form a group, and as hinted at in the discussion in the preceding paragraph, the group of deck transformations of the universal covering space of some topological space $X$ is exactly the fundamental group of $X$ .

More generally, a covering space for a topological space $X$ is another topological space $\tilde{X}$ with a continuous surjective map $p: \tilde{X}\rightarrow X$ such that the “inverse image” of a small neighborhood in $X$ is a disjoint union of small neighborhoods of $\tilde{X}$ . In the diagram above, the inverse image of the small neighborhood of $U$ of $X$ is the disjoint union of the small neighborhoods $S_{1}, S_{2}, S_{3}...$ of $\tilde{X}$ .

There are many possible covering spaces for a topological space. Here is another example for the circle (courtesy of user Pappus of Wikipedia):

We can think of this as a circle “covering” another circle. However, the first example above, the line covering the circle, is special. It is a universal covering space, which means that it is a covering space which is simply connected. The word “universal” however, means that this particular covering space also “covers” all the others.

Another example is the torus. Its universal covering space is the plane, and as we recall from The Moduli Space of Elliptic Curves, we can think of the torus as being obtained from the plane by dividing it into parallelograms using a lattice (which is also a group), and then identifying opposite edges of the parallelogram. Hence we can think of the torus as a quotient space (see Modular Arithmetic and Quotient Sets) obtained from the plane. The case of the circle and the line, which we have discussed earlier, is also very similar. Yet another example, which we have discussed in Rotations in Three Dimensions, is that of the $3$ -dimensional real projective space $\mathbb{RP}^{3}$ (which is also known in the theory of Lie groups as $\text{SO}(3)$ ), whose universal covering space is the $3$ -sphere $S^{3}$ (which is also known as $\text{SU}(2)$ ). Similar to the above examples, we can think of $\mathbb{RP}^{3}$ as a quotient space obtained from $S^{3}$ by identifying antipodal points (which are “opposite” points on the sphere which can be connected by a straight line passing through the center) on the sphere. From all these examples, we see that we can think of the universal covering space as being some sort of “unfolding” of the quotient space.

A perhaps more abstract way to think of the universal covering space is as the space whose points correspond to homotopy classes (see Homotopy Theory) of paths which start at a certain fixed basepoint (but is free to end on some other point). The set of these endpoints themselves correspond to the points of the topological space which is to be covered. However, we can get to the same endpoint through different paths which are not homotopic, i.e. they cannot be deformed into each other. If we construct a topological space whose points correspond to the homotopy classes of these paths, we will obtain a simply connected space, which is the universal covering space of our topological space.

We now go back to the definition of the fundamental group as the group of deck transformations of the universal covering space. Any covering space (of the same topological space) has its own group of deck transformations, and similar to how covering spaces can be covered by other covering spaces (and they are all covered by the universal covering space), the group of deck transformations of a covering space are also subgroups of the group of deck transformations of the covering space that covers the other covering space, and all the groups of deck transformations of covering spaces of the topological space are subgroups of the fundamental group (since it is the group of deck transformations of the universal covering space which covers all the other covering spaces of the topological space). In other words, the way that the covering spaces cover each other is reflected in the group structure of the fundamental group. This is reminiscent of the theory of Galois groups, where the group structure of the Galois group can shed light into the way certain fields are contained in other fields. This is the analogy mentioned earlier, and it has inspired many fruitful ideas in modern mathematics – for instance, it was one of the inspirations for the idea of the Grothendieck topos (see More Category Theory: The Grothendieck Topos).

References:

Fundamental group on Wikipedia

Covering Space on Wikipedia

Image by User Yonatan of Wikipedia

Image by User Pappus of Wikipedia

Coverings of the Circle on Youtube

Algebraic Topology by Allen Hatcher

A Concise Course in Algebraic Topology by J. P. May

Universal Covers on The Princeton Companion to Mathematics by Timothy Gowers, June Barrow-Green, and Imre Leader

Sheaves in Geometry and Logic: A First Introduction to Topos Theory by Saunders Mac Lane and Ieke Moerdijk

Some Useful Links on the Hodge Conjecture, Kahler Manifolds, and Complex Algebraic Geometry

April 5, 2017April 5, 2017 / Anton Hilado / 1 Comment

I’m going to be fairly busy in the coming days, so instead of the usual long post, I’m going to post here some links to interesting stuff I’ve found online (related to the subjects stated on the title of this post).

In the previous post, An Intuitive Introduction to String Theory and (Homological) Mirror Symmetry, we discussed Calabi-Yau manifolds (which are special cases of Kahler manifolds) and how their interesting properties, namely their Riemannian, symplectic, and complex aspects figure into the branch of mathematics called mirror symmetry, which is inspired by the famous, and sometimes controversial, proposal for a theory of quantum gravity (and more ambitiously a candidate for the so-called “Theory of Everything”), string theory.

We also mentioned briefly a famous open problem concerning Kahler manifolds called the Hodge conjecture (which was also mentioned in Algebraic Cycles and Intersection Theory). The links I’m going to provide in this post will be related to this conjecture.

As with the post An Intuitive Introduction to String Theory and (Homological) Mirror Symmetry, aside from introducing the subject itself, another of the primary intentions will be to motivate and explore aspects of algebraic geometry such as complex algebraic geometry, and their relation to other branches of mathematics.

Here is the page on the Hodge conjecture, found on the website of the Clay Mathematics Institute:

Hodge Conjecture on Clay Mathematics Institute

We have mentioned before that the Hodge conjecture is one of seven “Millenium Problems” for which the Clay Mathematics Institute is offering a million dollar prize. The page linked to above contains the official problem statement as stated by Pierre Deligne, and a link to a lecture by Dan Freed, which is intended for a general audience and quite understandable. The lecture by Freed is also available on Youtube:

Dan Freed on the Hodge Conjecture at the Clay Mathematics Institute on Youtube

Unfortunately the video of that lecture has messed up audio (although the lecture remains understandable – it’s just that the audio comes out of only one side of the speakers or headphones). Here is another set of videos by David Metzler on Youtube, which explains the Hodge conjecture (along with the other Millennium Problems) to a general audience:

Millennium Problem Talks on Youtube

The Hodge conjecture is also related to certain aspects of number theory. In particular, we have the Tate conjecture, which is another conjecture similar to the Hodge conjecture, but more related to Galois groups (see Galois Groups). Alex Youcis discusses it on the following post on his blog Hard Arithmetic:

The Tate Conjecture over Finite Fields on Hard Arithmetic

On the same blog there is also a discussion of a version of the Hodge conjecture called the $p$ -adic Hodge conjecture on the following post:

An Invitation to p-adic Hodge Theory; or How I Learned to Stop Worrying and Love Fontaine on Hard Arithmetic

The first part of the post linked to above discusses the Hodge conjecture in its classical form, while the second part introduces $p$ -adic numbers and related concepts, some aspects of which were discussed on this blog in Valuations and Completions.

A more technical discussion of the Hodge conjecture, Kahler manifolds, and complex algebraic geometry can be found in the following lecture of Claire Voisin, which is part of the Proceedings of the 2010 International Congress of Mathematicians in Hyderabad, India:

On the Cohomology of Algebraic Varieties by Claire Voisin

More about these subjects will hopefully be discussed on this blog at sometime in the future.

An Intuitive Introduction to String Theory and (Homological) Mirror Symmetry

April 1, 2017April 2, 2017 / Anton Hilado / 9 Comments

String theory is by far the most popular of the current proposals to unify the as of now still incompatible theories of quantum mechanics and general relativity. In this post we will give a short overview of the concepts involved in string theory, but not with the goal of discussing the theory itself in depth (hopefully there will be more posts in the future working towards this task). Instead, we will focus on introducing a very interesting and very beautiful branch of mathematics that arose out of string theory called mirror symmetry. In particular, we will focus on a version of it originally formulated by the mathematician Maxim Kontsevich in 1994 called homological mirror symmetry.

We will start with string theory. String theory started out as a theory of the nuclear forces that held together the protons and electrons in the nucleus of an atom. It was abandoned later on, due to a more successful theory called quantum chromodynamics taking its place. However, it was soon found out that string theory could model the elusive graviton, a particle “carrier” of gravity in the same way that a photon is a particle “carrier” of electromagnetism (the photon is more popularly referred to as a particle of light, but because light itself is an electromagnetic wave, it is also a manifestation of an electromagnetic field), and since then physicists have started developing string theory, no longer in the sole context of nuclear forces, but as a possible candidate for a working theory of quantum gravity.

The incompatibility of quantum mechanics and general relativity (which is currently our accepted theory of gravity) arises from the nonrenormalizability of gravity. In calculations in quantum field theory (see Some Basics of Relativistic Quantum Field Theory and Some Basics of (Quantum) Electrodynamics), there appear certain “nonsensical” quantities which are made sense of via a “corrective” procedure called renormalization (not to be confused with some other procedures called “normalization”). While the way that renormalization works is not really completely understood at the moment, it is known that this procedure at least “works” – this means that it produces the correct values of quantities, as can be checked via experiment.

Renormalization, while it works for the other forces, however fails for gravity. Roughly this is sometimes described as gravity “wildly fluctuating” at the smallest scales. What we know is that this signals, for us, a lack of knowledge of what physics is like at these extremely small scales (much smaller than the current scale of quantum mechanics).

String theory attempts to solve this conundrum by proposing that particles, at the very smallest scales, are not “particles” at all, but “strings”. This takes care of the problem of fluctuations at the smallest scales, since there is a limit to how small the scale can be, set by the length of the strings. It is perhaps worth noting at this point that the next most popular contender to string theory, loop quantum gravity, tackles this problem by postulating that space itself is not continuous, but “discretized” into units of a certain length. For both theories, this length is predicted to be around $10^{-35}$ meters, a constant quantity which is known as the Planck length.

Over time, as string theory was developed, it became more ambitious, aiming to provide not only the unification of quantum mechanics and general relativity, but also the unification of the four fundamental forces – electromagnetism, the weak nuclear force, the strong nuclear force, and gravity, under one “theory of everything“. At the same time, it needed more ingredients – to be able to account for bosons, the particles carrying “forces”, such as photons and gravitons, and the fermions, particles that make up matter, such as electrons, protons, and neutrons, a new ingredient had to be added, called supersymmetry. In addition, it worked not in the four dimensions of spacetime that we are used to, but instead required ten dimensions (for the “bosonic” string theory, before supersymmetry, the number of dimensions required was a staggering twenty-six)!

How do we explain spacetime having ten dimensions, when we experience only four? It turns out, even before string theory, the idea of extra dimensions was already explored by the physicists Theodor Kaluza and Oskar Klein. They proposed a theory unifying electromagnetism and gravity by postulating an “extra” dimension which was “curled up” into a loop so small we could never notice it. The usual analogy is that of an ant crossing a wire – when the radius of the wire is big, the ant realizes that it can go sideways along the wire, but when the radius of the wire is small, it is as if there is only one dimension that the ant can move along.

So we now have this idea of six curled up dimensions of spacetime, in addition to the usual four. It turns out that there are so many ways that these dimensions can be curled up. This phenomenon is called the string theory landscape, and it is one of the biggest problems facing string theory today. What could be the specific “shape” in which these dimensions are curled up, and why are they not curled up in some other way? Some string theorists answer this by resorting to the controversial idea of a multiverse, so that there are actually several existing universes, each with its own way of how the extra six dimensions are curled up, and we just happen to be in this one because, perhaps, this is the only one where the laws of physics (determined by the way the dimensions are curled up) are able to support life. This kind of reasoning is called the anthropic principle.

In addition to the string theory landscape, there was also the problem of having several different versions of string theory. These problems were perhaps alleviated by the discovery of mysterious dualities. For example, there is the so-called T-duality, where a compactification (a “curling up”) with a bigger radius gives the same laws of physics as a compactification with a smaller, “reciprocal” radius. Not only do the concept of dualities connect the different ways in which the extra dimensions are curled up, they also connect the several different versions of string theory! In 1995, the physicist Edward Witten conjectured that this is perhaps because all these different versions of string theory come from a single “mother theory”, which he called “M-theory“.

In 1991, physicists Philip Candelas, Xenia de la Ossa, Paul Green, and Linda Parkes used these dualities to solve a mathematical problem that had occupied mathematicians for decades, that of counting curves on a certain manifold (a manifold is a shape without sharp corners or edges) known as a Calabi-Yau manifold. In the context of Calabi-Yau manifolds, which are some of the shapes in which the extra dimensions of spacetime are postulated to be curled up, these dualities are known as mirror symmetry. With the success of Candelas, de la Ossa, Green, and Parkes, mathematicians would take notice of mirror symmetry and begin to study it as a subject of its own.

Calabi-Yau manifolds are but special cases of Kahler manifolds, which themselves are very interesting mathematical objects because they can be studied using three aspects of differential geometry – Riemannian geometry, symplectic geometry, and complex geometry.

We have already encountered examples of Kahler manifolds on this blog – they are the elliptic curves (see Elliptic Curves and The Moduli Space of Elliptic Curves). In fact elliptic curves are not only Kahler manifolds but also Calabi-Yau manifolds, and they are the only two-dimensional Calabi-Yau manifolds (we sometimes refer to them as “one-dimensional” when we are considering “complex dimensions”, as is common practice in algebraic geometry – this apparent “discrepancy” in counting dimensions arises because we need two real numbers to specify a complex number). In string theory of course we consider six-dimensional (three-dimensional when considering complex dimensions) Calabi-Yau manifolds, since there are six extra curled up dimensions of spacetime, but often it is also fruitful to study also the other cases, especially the simpler ones, since they can serve as our guide for the study of the more complicated cases.

Riemannian geometry studies Riemannian manifolds, which are manifolds equipped with a metric tensor, which intuitively corresponds to an “infinitesimal distance formula” dependent on where we are on the manifold. We have already encountered Riemannian geometry before in Geometry on Curved Spaces and Connection and Curvature in Riemannian Geometry. There we have seen that Riemannian geometry is very important in the mathematical formulation of general relativity, since in this theory gravity is just the curvature of spacetime, and the metric tensor expresses this curvature by showing how the formula for the infinitesimal distance between two points (actually the infinitesimal spacetime interval between two events) changes as we move around the manifold.

Symplectic geometry, meanwhile, studies symplectic manifolds. If Riemannian manifolds are equipped with a metric tensor that measures “distances”, symplectic manifolds are equipped with a symplectic form that measures “areas”. The origins of symplectic geometry are actually related to William Rowan Hamilton’s formulation of classical mechanics (see Lagrangians and Hamiltonians), as developed later on by Henri Poincare. There the object of study is phase space, which gives the state of a system based on the position and momentum of the objects that comprise it. It is this phase space that is expressed as a symplectic manifold.

Complex geometry, following our pattern, studies complex manifolds. These are manifolds which locally look like $\mathbb{C}^{n}$ , in the same way that ordinary differentiable manifolds locally look like $\mathbb{R}^{n}$ . Just as Riemannian geometry has metric tensors and symplectic geometry has symplectic forms, complex geometry has complex structures, mappings of tangent spaces with the property that applying them twice is the same as multiplication by $-1$ , mimicking the usual multiplication by the imaginary unit $i$ on the complex plane.

Complex manifolds are not only part of differential geometry, they are also often studied using the methods of algebraic geometry! We recall (see Basics of Algebraic Geometry) that algebraic geometry studies varieties and schemes, which are shapes such as lines, conic sections (parabolas, hyperbolas, ellipses, and circles), and elliptic curves, that can be described by polynomials (their modern definitions are generalizations of this concept). In fact, all Calabi-Yau manifolds can be described by polynomials, such as the following example, due to user Andrew J. Hanson of Wikipedia:

This is a visualization (actually a sort of “cross section”, since we can only display two dimensions and this object is actually six-dimensional) of the Calabi-Yau manifold described by the following polynomial equation:

$\displaystyle V^{5}+W^{5}+X^{5}+Y^{5}+Z^{5}=0$

This polynomial equation (known as the Fermat quintic) actually describes the Calabi-Yau manifold in projective space using homogeneous coordinates. This means that we are using the concepts of projective geometry (see Projective Geometry) to include “points at infinity“.

We note at this point that Kahler manifolds and Calabi-Yau manifolds are interesting in their own right, even outside of the context of string theory. For instance, we have briefly mentioned in Algebraic Cycles and Intersection Theory the Hodge conjecture, one of seven “Millenium Problems” for which the Clay Mathematics Institute is currently offering a million-dollar prize, and it concerns Kahler manifolds. Perhaps most importantly, it “unifies” several different branches of mathematics; as we have already seen, the study of Kahler manifolds and Calabi-Yau manifolds involves Riemannian geometry, symplectic geometry, complex geometry, and algebraic geometry. The more recent version of mirror symmetry called homological mirror symmetry further adds category theory and homological algebra to the mix.

Now what mirror symmetry more specifically states is that a version of string theory called Type IIA string theory, on a spacetime with extra dimensions compactified onto a certain Calabi-Yau manifold $V$ , is the same as another version of string theory, called Type IIB string theory, on a spacetime with extra dimensions compactified onto another Calabi-Yau manifold $W$ , which is “mirror” to the Calabi-Yau manifold $V$ .

The statement of homological mirror symmetry (which is still conjectural, but mathematically proven in certain special cases) expresses the idea of the previous paragraph as follows (quoted verbatim from the paper Homological Algebra of Mirror Symmetry by Maxim Kontsevich):

Let $(V,\omega)$ be a $2n$ -dimensional symplectic manifold with $c_{1}(V)=0$ and $W$ be a dual $n$ -dimensional complex algebraic manifold.

The derived category constructed from the Fukaya category $F(V)$ (or a suitably enlarged one) is equivalent to the derived category of coherent sheaves on a complex algebraic variety $W$ .

The statement makes use of the language of category theory and homological algebra (see Category Theory, More Category Theory: The Grothendieck Topos, Even More Category Theory: The Elementary Topos, Exact Sequences, More on Chain Complexes, and The Hom and Tensor Functors), but the idea that it basically expresses is that there exists a relation between the symplectic aspects of the Calabi-Yau manifold $V$ , as encoded in its Fukaya category, and the complex aspects of the Calabi-Yau manifold $W$ , as encoded in its category of coherent sheaves (see Sheaves and More on Sheaves). As we have said earlier, the subjects of algebraic geometry and complex geometry are closely related, and hence the language of sheaves show up in (and is an important part of) both subjects. The concept of derived categories, which generalize derived functors like the Ext and Tor functors, allow us to relate the two categories, which otherwise would be expressing different concepts. Inspired by string theory, therefore, we have now a deep and beautiful idea in geometry, relating its different aspects.

Is string theory the correct way towards a complete theory of quantum gravity, or the so-called “theory of everything”? As of the moment, we don’t know. Quantum gravity is a very difficult problem, and the scales involved are still far out of our reach – in order to probe smaller and smaller scales we need particle accelerators with higher and higher energies, and right now the technologies that we have are still very, very far from the scales which are relevant to quantum gravity. Still, it is hoped for that whatever we find in experiments in the near future, not only in the particle accelerators but also in the radio telescopes that look out into space, will at least guide us towards the correct path.

There are some who believe that, in the absence of definitive experimental evidence, mathematical beauty is our next best guide. And, without a doubt, string theory is related to, and has inspired, some very beautiful and very interesting mathematics, including that which we have discussed in this post. Still, physics, like all natural science, is empirical (based on evidence and observation), and hence it is ultimately physical evidence that will be the judge of correctness. It may yet turn out that string theory is wrong, and that it is a different theory which describes the fundamental physical laws of nature, or that it needs drastic modifications to its ideas. This will not invalidate the mathematics that we have described here, anymore than the discoveries of Copernicus invalidated the mathematics behind the astronomical model of Ptolemy – in fact this mathematics not only outlived the astronomy of Ptolemy, but served the theories of Copernicus, and his successors, just as well. Hence we cannot really say that the efforts of Ptolemy were wasted, since even though his scientific ideas were shown to be wrong, still his mathematical methods were found very useful by those who succeeded him. Thus, while our current technological limitations prohibit us from confirming or ruling out proposals for a theory of quantum gravity such as string theory, there is still much to be gained from such continued efforts on the part of theory, while experiment is still in the process of catching up.

Our search for truth continues. Meanwhile, we have beauty to cultivate.

References:

String Theory on Wikipedia

Mirror Symmetry on Wikipedia

Homological Mirror Symmetry on Wikipedia

Calabi-Yau Manifold on Wikipedia

Kahler Manifold on Wikipedia

Riemannian Geometry on Wikipedia

Symplectic Geometry on Wikipedia

Complex Geometry on Wikipedia

Fukaya Category on Wikipedia

Coherent Sheaf on Wikipedia

Derived Category on Wikipedia

Image by User Andrew J. Hanson of Wikipedia

Homological Algebra of Mirror Symmetry by Maxim Kontsevich

The Elegant Universe: Superstrings, Hidden Dimensions, and the Quest for the Ultimate Theory by Brian Greene

String Theory by Joseph Polchinski

String Theory and M-Theory: A Modern Introduction by Katrin Becker, Melanie Becker, and John Schwarz

Differential Forms

March 28, 2017March 29, 2017 / Anton Hilado / 3 Comments

Differential forms are important concepts in differential geometry and mathematical physics. For example, they can be used to express Maxwell’s equations (see Some Basics of (Quantum) Electrodynamics) in a very elegant form. In this post, however, we will introduce these mathematical objects as generalizing certain aspects of integral calculus (see An Intuitive Introduction to Calculus), allowing us to perform integration over surfaces, volumes, or their higher-dimensional analogues.

We recall from An Intuitive Introduction to Calculus the statement of the fundamental theorem of calculus:

$\displaystyle \int_{a}^{b}\frac{df}{dx}dx=f(b)-f(a)$ .

Regarding the left hand side of this equation, we usually we say that we integrate over the interval from $a$ to $b$ ; we may therefore also write it more suggestively as

$\displaystyle \int_{[a,b]}\frac{df}{dx}dx=f(b)-f(a)$ .

We note that $a$ and $b$ form the boundary of the interval $[a,b]$ . We denote the boundary of some “shape” $M$ by $\partial M$ . Therefore, in this case, $\partial [a,b]=\{a\}\cup\{b\}$ .

Next we are going to perform some manipulations on the notation, which, while we will not thoroughly justify in this post, are meant to be suggestive and provide intuition for the discussion on differential forms. First we need the notion of orientation. We can imagine, for example, an “arrow” pointing from $a$ to $b$ ; this would determine one orientation. Another would be determined by an “arrow” pointing from $b$ to $a$ . This is important because we need a notion of integration “from $a$ to $b$ ” or “from $b$ to $a$ “, and the two are not the same. In fact,

$\displaystyle \int_{a}^{b}\frac{df}{dx}dx=-\int_{b}^{a}\frac{df}{dx}dx$

i.e. there is a change of sign if we “flip” the orientation. Although an interval such as $[a,b]$ is one-dimensional, the notion of orientation continues to make sense in higher dimension. If we have a surface, for example, we may consider going “clockwise” or “counterclockwise” around the surface. Alternatively we may consider an “arrow” indicating which “side” of the surface we are on. For three dimensions or higher it is harder to visualize, but we will be able to make this notion more concrete later on with differential forms.

Given the notion of orientation, let us now denote the boundary of the interval $[a,b]$ , taken with orientation, for instance, “from $a$ to $b$ “, by $\{a\}^{-}\cup\{b\}^{+}$ .

Let us now write

$\displaystyle \frac{df}{dx}dx=df$

and then we can write the fundamental theorem of calculus as

$\displaystyle \int_{[a,b]}df=f(b)-f(a)$ .

Then we consider the idea of “integration over points”, by which we refer to simply evaluating the function at those points, with the orientation taken into account, such that we have

$\displaystyle \int_{\{a\}^{-}\cup\{b\}^{+}}f=f(b)-f(a)$

Recalling that $\partial [a,b]=\{a\}^{-}\cup\{b\}^{+}$ , this now gives us the following expression for the fundamental theorem of calculus:

$\displaystyle \int_{[a,b]}df=\int_{\{a\}^{-}\cup\{b\}^{+}}f$

$\displaystyle \int_{[a,b]}df=\int_{\partial [a,b]}f$

Things may still be confusing to the reader at this point – for instance, that integral on the right hand side looks rather weird – we will hopefully make things more concrete shortly. For now, the rough idea that we want to keep in mind is the following:

The integral of a “differential” of some function over some shape is equal to the integral of the function over the boundary of the shape.

In one dimension, this is of course the fundamental theorem of calculus as we have stated it earlier. For two dimensions, there is a famous theorem called Green’s theorem. In three dimensions, there are two manifestations of this idea, known as Stokes’ theorem and the divergence theorem. The more “concrete” version of this statement, which we want to discuss in this post, is the following:

The integral of the exterior derivative of a differential form over a manifold with boundary is equal to the integral of the differential form over the boundary.

We now discuss what these differential forms are. Instead of the formal definitions, we will start with special cases, develop intuition with examples, and attempt to generalize. The more formal definitions will be left to the references. We will start with the so-called $1$ -forms, which are “linear combinations” of the “differentials”.

We can think of these “differentials” as merely symbols for now, or perhaps consider them analogous to “infinitesimal quantities” in calculus. In differential geometry, however, they are actually “dual” to vectors, mapping vectors to numbers in the same way that row matrices map column matrices to the numbers which serve as their scalars (see Matrices) of the coordinates, with coefficients which are functions:

$\displaystyle f_{1}dx+f_{2}dy+f_{3}dz$

From now on, to generalize, instead of the coordinates $x$ , $y$ , and $z$ we will use $x^{1}$ , $x^{2}$ , $x^{3}$ , and so on. We will write exponents as $(x^{1})^{2}$ , to hopefully avoid confusion.

From these $1$ -forms we can form $2$ -forms by taking the wedge product. In ordinary multivariable calculus, the following expression

$\displaystyle dxdy$

represents an “infinitesimal area”, and so for example the integral

$\displaystyle \int_{0}^{1}\int_{0}^{1}dxdy$

gives us the area of a square with vertices at $(0,0)$ , $(1,0)$ , $(0,1)$ , and $(1,1)$ . The wedge product expresses this same idea (in fact the wedge product $dx\wedge dy$ is often also called the area form, mirroring the idea expressed by $dxdy$ earlier), except that we want to include the concept of orientation that we discussed earlier. Therefore, in order to express this idea of orientation, we require the wedge product to satisfy the following property called antisymmetry:

$\displaystyle dx^{1}\wedge dx^{2}=-dx^{2}\wedge dx^{1}$

Note that antisymmetry implies the following relation:

$\displaystyle dx^{i}\wedge dx^{i}=-dx^{i}\wedge dx^{i}$

$\displaystyle dx^{i}\wedge dx^{i}=0$

In other words, the wedge product of such a differential form with itself is equal to zero.

We can also form $3$ -forms, $4$ -forms, etc. using the wedge product. The collection of all these $n$ -forms, for every $n$ , is the algebra of differential forms. This means that we can add, subtract, and form wedge products of differential forms. Ordinary functions themselves form the $0$ -forms.

We can also take what is called the exterior derivative of differential forms. If, for example, we have a differential form $\omega$ given by the following expression,

$\displaystyle \omega=f dx^{a}$

then the exterior derivative of $\omega$ , written $d\omega$ , is given by

$\displaystyle d\omega=\sum_{i=1}^{n}\frac{\partial f}{\partial x_{i}}dx^{i}\wedge dx^{a}$ .

We note that the exterior derivative of a $n$ -form is an $n+1$ -form. We also note that the exterior derivative of an exterior derivative is always zero, i.e. $d(d\omega)=0$ for any differential form $\omega$ . A differential form which is the exterior derivative of some other differential form is called exact. A differential form whose exterior derivative is zero is called closed. The statement $d(d\omega)=0$ can also be expressed as follows:

All exact forms are closed.

However, not all closed forms are exact. This is reminiscent of the discussion in Homology and Cohomology, and in fact the study of closed forms which are not exact leads to the theory of de Rham cohomology, which is a very important part of modern mathematics and mathematical physics.

Given the idea of the exterior derivative, the general form of the fundamental theorem of calculus is now given by the generalized Stokes’ theorem (sometimes simply called the Stokes’ theorem; historically however, as alluded to earlier, the original Stokes’ theorem only refers to a special case in three dimensions):

$\displaystyle \int_{M}d\omega=\int_{\partial M}\omega$

This is the idea we alluded to earlier, relating the integral of a differential form (which includes functions as $0$ -forms) over some “shape” to the integral of the exterior derivative of the differential form over the boundary of that “shape”.

There is much more to the theory of differential forms than we have discussed here. For example, although we have referred to these “shapes” as manifolds with boundary, more generally they are “chains” (see also Homology and Cohomology – the similarities are not coincidental!). There are restrictions on these chains in order for the integral to give a function; for example, an $n$ -form must be integrated over an $n$ -dimensional chain (or simply $n$ -chain) to give a function, otherwise they will give some other differential form. An $m$ -form integrated over an $n$ -chain gives an $m-n$ form. Also, more rigorously the concept of integration on more complicated spaces involves the notion of “pullback”. We will leave these concepts to the references for now, contenting ourselves with the discussion of the wedge product and exterior derivative in this post. The application of differential forms to physics is discussed in the very readable book Gauge Fields, Knots and Geometry by John Baez and Javier P. Muniain.

References:

Differential Forms on Wikipedia

Green’s Theorem on Wikipedia

Divergence Theorem on Wikipedia

Stokes’ Theorem on Wikipedia

De Rham Cohomology on Wikipedia

Calculus on Manifolds by Michael Spivak

Gauge Fields, Knots and Gravity by John Baez and Javier P. Muniain

Geometry, Topology, and Physics by Mikio Nakahara

Simplices

March 18, 2017 / Anton Hilado / 1 Comment

In Homology and Cohomology, we showed how to study the topology of spaces using homology and cohomology groups, which are obtained via the construction of chain complexes out of abelian groups of subspaces of the topological space. However, we have not elaborated how this is achieved. In this post we introduce the notion of a simplex, which gives us a method of “triangulating” the space so that we can have construct the chains that make up our chain complex.

An $n$ -simplex can be thought of as the $n$ -dimensional analogue of a triangle. More technically, it is the smallest convex set in some Euclidean space $\mathbb{R}^{m}$ containing $n+1$ points $v_{0},...,v_{n}$ , called its vertices, such that the “difference vectors” defined by $v_{1}-v_{0},...,v_{n}-v_{0}$ are linearly independent. We will use the notation $[v_{0},...,v_{n}]$ to denote a simplex. We will keep track of the ordering of the vertices of the simplex, and we will always make use of the convention that the subscripts indexing the vertices are to be written in increasing order.

To make things more concrete, we discuss one of the most basic examples of an $n$ -simplex, the standard $n$ -simplex. It is defined to be the subset of $n+1$ -dimensional Euclidean space $\mathbb{R}^{n+1}$ given by

$\displaystyle \Delta^{n}=\{(t_{0},...,t_{n})\in\mathbb{R}^{n+1}|\sum_{i=0}^{n}t_{i}=1\text{ and }t_{i}\geq 0\text{ for all }i\}$

The standard $0$ -simplex is a point (actually the point $x=1$ on the real line $\mathbb{R}$ ), the standard $1$ -simplex is a line segment connecting the points $(1,0)$ and $(0,1)$ in the $x$ – $y$ plane, the standard $2$ -simplex is a triangle (including its interior) whose vertices are located at $(1,0,0)$ , $(0,1,0)$ , and $(0,0,1)$ in $3$ -dimensional Euclidean space, and the standard $3$ -simplex is a tetrahedron (again including its interior) whose vertices are located at $(1,0,0,0)$ , $(0,1,0,0)$ , $(0,0,1,0)$ , and $(0,0,0,1)$ in $4$ -dimensional Euclidean space. The standard higher-dimensional simplices have analogous descriptions. Here is an image depicting the standard $2$ -simplex, courtesy of user Tosha of Wikipedia:

Consider now a $2$ -simplex $[v_{0},v_{1},v_{2}]$ . This is of course a triangle. To this $2$ -simplex there are three related $1$ -simplices, namely $[v_{0},v_{1}]$ , $[v_{1},v_{2}]$ , and $[v_{0},v_{2}]$ . They can be thought of as the “edges” of the $2$ -simplex $[v_{0},v_{1},v_{2}]$ , and together they form the boundary of the $2$ -simplex, written $\partial [v_{0},v_{1},v_{2}]$ .

We want to use the concept of simplices in order to construct chains, which in turn form chain complexes, so that we can make use of the techniques of homology and cohomology. Crucial to the notion of chains is the abelian group structure, so that we can “add” and “subtract” $n$ -chains, for a given $n$ , to form new $n$ -chains. This abelian group structure will also help us in making the idea of a boundary of a simplex more concrete, and at the same time provide us with an explicit expression for the boundary operator (also known as the boundary map, or boundary function) also crucial to the idea of a chain complex and its homology. This boundary operator (written $\partial$ ) is given by

$\displaystyle \partial [v_{0},...,v_{n}]=\sum_{i}^{n}(-1)^{i}[v_{0},...,\hat{v_{i}},...,v_{n}]$

where $\hat{v_{i}}$ means that the vertex $v_{i}$ is to be omitted. Therefore, for the $2$ -simplex $[v_{0},v_{1},v_{2}]$ , our boundary $\partial[v_{0},v_{1},v_{2}]$ is given by

$\displaystyle \partial [v_{0},v_{1},v_{2}]=[v_{0},v_{1}]-[v_{0},v_{2}]+[v_{1},v_{2}]$ .

Simplices, with the boundary operators, can therefore be used to form chain complexes. The chains in this chain complex consist of “linear combinations” of simplices. We can then apply the notions of cycles and boundaries, and the principle that a space that is the boundary of another space has itself no boundary (but not all spaces that have no boundaries are the boundaries of other spaces – this is what the homology groups express), to study topology.

Of course, not all spaces look like simplices. But for many spaces, we can always map simplices to them via a homoeomorphism. Intuitively, this corresponds to “triangulating” the space. For example, we may map the boundary of a tetrahedron (made up of four triangles – $2$ -simplices – with certain edges and vertices in common) homeomorphically onto the sphere. What this means is that we can essentially then take the techniques that we have developed for the tetrahedron and apply them to the sphere.

Similarly, we can take a square with a diagonal (made up of two $2$ -simplices, again with certain edges and vertices in common), identify opposite edges of the boundary, and map it homeomorphically to the torus. This allows us to calculate the homology groups of the torus.

The use of simplices to construct chain complexes for taking the homology groups of a topological space is called simplicial homology. A generalization that involves maps that may not be homeomorphic is called singular homology. There are also other ways to construct chain complexes, for instance, we also have cellular cohomology, which instead makes use of of “cells” instead of simplices. Just as simplices are generalizations of triangles and tetrahedrons, cells are generalizations of discs and balls. A space made up of simplices is called a simplicial complex, while a space made up of cells is called a CW-complex.

Aside from algebraic topology in the usual sense, the notion of simplices are also useful in higher category theory. We recall from Category Theory that a category is made up of objects and morphisms, sometimes also called arrows, between these objects. In higher category theory, we also consider “morphisms between morphisms”, “morphisms between morphisms between morphisms”, and so on. This is reminiscent of simplices, in which we have vertices, edges, faces, and higher-dimensional analogues. Hence, the idea of simplices can be abstracted so that they can be used for the constructions of higher category theory. This leads to the theory of simplicial categories.

References:

Simplex on Wikipedia

Simplicial Complex on Wikipedia

Simplicial Homology on Wikipedia

Singular Homology on Wikipedia

Higher Category Theory on Wikipedia

Image by User Tomruen of Wikipedia (Image Created by Robert Webb’s Stella Software)

Image by User Tosha of Wikipedia

Algebraic Topology by Allen Hatcher

A Concise Course in Algebraic Topology by J. P. May

Vector Fields, Vector Bundles, and Fiber Bundles

January 6, 2017May 31, 2017 / Anton Hilado / 8 Comments

In physics we have the concept of a vector field. Intuitively, a vector field is given by specifying a vector (in the sense of a quantity with magnitude and direction) at every point in a certain “space”. For instance, the wind velocity on the surface of our planet is a vector field. If we neglect the upward or downward dimension, and look only at the northward, southward, eastward, and westward directions, we have what we usually see on weather maps on the news. In one city the wind might be blowing strongly to the north, in another city it might be blowing weakly to the east, and in a third city it might be blowing moderately to the southwest.

If, instead of specifying a vector space (see Vector Spaces, Modules, and Linear Algebra) at every point, instead of just a single vector, we obtain instead the concept of a vector bundle. Given a vector bundle, we can obtain a vector field by choosing just one vector in the vector space. More technically, we say that a vector field is a section of the vector bundle.

A vector space can be thought of as just a certain kind of space; in our example of wind velocities on the surface of the Earth, the vector space that we attach to every point is the plane $\mathbb{R}^{2}$ endowed with an intuitive vector space structure. Given a point on the plane, we draw an “arrow” with its “tail” at the chosen origin of the plane and its “head” at the given point. We can then add and scale these arrows to obtain other arrows, hence, these arrows form a vector space. This “graphical” method of studying vectors (again in the sense of a quantity with magnitude and direction) is in fact one of the most common ways of introducing the concept of vectors in physics.

If, instead of a vector space such as the plane $\mathbb{R}^{2}$ we generalize to other kinds of spaces such as the circle $S^{1}$ , we obtain the notion of a fiber bundle. A vector space is therefore just a special case of a fiber bundle. In Category Theory, we described the torus as a fiber bundle, obtained by “gluing” a circle to every point of another circle. The shape that is glued is called the “fiber“, and the shape to which the fibers are glued is called the “base“.

Simply gluing spaces to the points of another space does not automatically mean that the space obtained is a fiber bundle, however. There is another requirement. Consider, for example, a cylinder. This can be described as a fiber bundle, with the fibers given by lines, and the base given by a circle (this can also be done the other way around, but we use this description for the moment because we will use it to describe an important condition for a space to be a fiber bundle). However, another fiber bundle can be obtained from lines (as the fibers) and a circle (as the base). This other fiber bundle can be obtained by “twisting” the lines as we “glue” them to the points of a circle, resulting in the very famous shape known as the Mobius strip.

The cylinder, which exhibits no “twisting”, is the simplest kind of fiber bundle, called a trivial bundle. Still, even if the Mobius strip has some kind of “twisting”, if we look at them “locally”, i.e. only on small enough areas, there is no difference between the cylinder and the Mobius strip. It is only when we look at them “globally” that we can distinguish the two. This is the important requirement for a space to be a fiber bundle. Locally, they must “look like” the trivial bundle. This condition is related to the notion of continuity (see Basics of Topology and Continuous Functions).

The concept of fiber bundles can be found everywhere in physics, and forms the language for many of its branches. We have already stated an example, with vector fields on a space. Aside from wind velocities (and the velocities of other fluids), the concept of vector fields are also used to express quantities such as electric and magnetic fields.

Fiber bundles can also be used to express ideas that are not so easily visualized. For example, in My Favorite Equation in Physics we mentioned the concept of a phase space, whose coordinates represent the position and momentum of a system, which is used in the Hamiltonian formulation of classical mechanics. The phase space of a system is an example of a kind of fiber bundle called a cotangent bundle. Meanwhile, in Einstein’s general theory of relativity, the concept of a tangent bundle is used to study the curvature of spacetime (which in the theory is what we know as gravity, and is related to mass, or more generally, energy and momentum).

More generally, the tangent bundle can be used to study the curvature of objects aside from spacetime, including more ordinary objects like a sphere, or hills and valleys on a landscape. This leads to a further generalization of the notion of “curvature” involving other kinds of fiber bundles aside from tangent bundles. This more general idea of curvature is important in the study of gauge theories, which is an important part of the standard model of particle physics. A good place to start for those who want to understand curvature in the context of tangent bundles and fiber bundles is by looking up the idea of parallel transport.

Meanwhile, in mathematics, fiber bundles are also very interesting in their own right. For example, vector bundles on a space can be used to study the topology of a space. One famous result involving this idea is the “hairy ball theorem“, which is related to the observation that on our planet every typhoon must have an “eye”. However, on something that is shaped like a torus instead of a sphere (like, say, a space station with an artificial atmosphere), one can have a typhoon with no eye, simply by running the wind along the walls of the torus. Replacing wind velocities by magnetic fields, this becomes the reason why fusion reactors that use magnetic fields to contain the very hot plasma are shaped like a torus instead of like a sphere. We recall, of course, that the sphere and the torus are topologically inequivalent, and this is reflected in the very different characteristics of vector fields on them.

The use of vector bundles in topology has led to such subjects of mathematics such as the study of characteristic classes and K-theory. The concept of mathematical objects “living on” spaces should be reminiscent of the ideas discussed in Presheaves and Sheaves; in fact, in algebraic geometry the two ideas are very much related. Since algebraic geometry serves as a “bridge” between ideas from geometry and ideas from abstract algebra, this then leads to the subject called algebraic K-theory, where ideas from topology get carried over to abstract algebra and linear algebra (even number theory).

References:

Fiber Bundle on Wikipedia

Vector Bundle on Wikipedia

Vector Field on Wikipedia

Parallel Transport on Wikipedia

What is a Field? at Rationalizing the Universe

Algebraic Geometry by Andreas Gathmann

Algebraic Topology by Allen Hatcher

A Concise Course in Algebraic Topology by J. P. May

Geometrical Methods of Mathematical Physics by Bernard F. Schutz

Geometry, Topology and Physics by Mikio Nakahara

Exact Sequences

December 21, 2016April 4, 2017 / Anton Hilado / 4 Comments

In Homology and Cohomology we introduced the idea of chain complexes to help us obtain information about topological spaces. We recall that a chain complex is made up of abelian groups of spaces $C_{n}$ and boundary homomorphisms $\partial_{n}: C_{n}\rightarrow C_{n-1}$ such that for all $n$ the composition of successive boundary homomorphisms $\partial_{n-1}\circ \partial_{n}: C_{n}\rightarrow C_{n-2}$ sends every element in $C_{n}$ to the zero element in $C_{n-2}$ .

Chain complexes can be expressed using the following diagram:

$...\xrightarrow{\partial_{n+3}}C_{n+2}\xrightarrow{\partial_{n+2}}C_{n+1}\xrightarrow{\partial_{n+1}}C_{n}\xrightarrow{\partial_{n}}C_{n-1}\xrightarrow{\partial_{n-1}}C_{n-2}\xrightarrow{\partial_{n-2}}...$

We now abstract this idea, generalizing it so that the groups $C_{n}$ do not necessarily have to be topological spaces, and show an example of a chain complex that is ubiquitous in mathematics.

First we recall some ideas from Homology and Cohomology. Our “important principle” was summarized in the following statement:

All boundaries are cycles.

Boundaries in $C_{n}$ are elements of the image of the boundary homomorphism $\partial_{n+1}$ . Cycles in $C_{n}$ are elements of the kernel of the boundary homomorphism $\partial_{n}$ . Therefore, we can also state our “important principle” as follows:

$\text{Im }\partial_{n+1}\subseteq \text{Ker }\partial_{n}$ for all $n$

This is of course just another restatement of the defining property of all chain complexes that two successive boundary functions when composed send every element of its domain to the zero element of its range.

There is an important kind of chain complex with the following property:

$\text{Im }\partial_{n+1}=\text{Ker }\partial_{n}$ for all $n$

Such a chain complex is called an exact sequence. Sometimes we just say that the chain complex is exact. We will show some simple examples of exact sequences, but for these examples we will drop the notation of the boundary homomorphism $\partial_{n}$ to show that many properties of ordinary functions can be expressed in terms of exact sequences.

Consider, for example, abelian groups $A$ , $B$ , and $C$ . The identity elements of $A$ , $B$ , and $C$ will be denoted by $0$ , writing $0\in A$ , $0\in B$ , and $0\in C$ if necessary. We will also write $0$ to denote the trivial abelian group consisting only of the single element $0$ . Let us now look at the exact sequence

$0\rightarrow A\xrightarrow{f} B$

where $0\rightarrow A$ is the inclusion function sending $0\in 0$ to $0\in A$ . The image of this inclusion function is therefore $0\in A$ . By the defining property of exact sequences, this is also the kernel of the function $f:A\rightarrow B$ . In other words, $f$ sends $0\in A$ to $0\in B$ . It is a property of group homomorphisms that whenever the kernel consists of only one element, the homomorphism is an injective, or one-to-one, function. This means that no more than one element of the domain gets sent to the same element in the range. Since this function is also a homomorphism, it is also called a monomorphism.

Meanwhile, let us also consider the exact sequence

$B\xrightarrow{g} C\rightarrow 0$

where $C\rightarrow 0$ is the “constant” function that sends any element in $C$ to $0$ . The kernel of this constant function is therefore the entirety of $C$ . By the defining property of exact sequences, this is also the image of the function $B\rightarrow C$ . In other words, the image of the function $g$ is the entirety of $C$ , or we can also say that every element of $C$ is assigned by $g$ to some element of $B$ . Such a function is called surjective, or onto. Since this function is also a homomorphism, it is also called an epimorphism.

The exact sequence

$0\rightarrow A\xrightarrow{f} B\xrightarrow{g} C\rightarrow 0$

is important in many branches of mathematics, and is called a short exact sequence. This means that $f$ is a monomorphism, $g$ is an epimorphism, and that $\text{im }f=\text{ker g}$ in $B$ . As an example of a short exact sequence of abelian groups, we have

$0\rightarrow 2\mathbb{Z}\xrightarrow{f} \mathbb{Z}\xrightarrow{g} \mathbb{Z}/2\mathbb{Z}\rightarrow 0$

(see also Modular Arithmetic and Quotient Sets). The monomorphism $f$ takes the abelian group of even integers $2\mathbb{Z}$ and “embeds” them into the abelian group of the integers $\mathbb{Z}$ . The epimorphism $g$ then sends the integers in $\mathbb{Z}$ to the element $0$ in $\mathbb{Z}/2\mathbb{Z}$ if they are even, and to the element $1$ in $\mathbb{Z}/2\mathbb{Z}$ if they are odd. We see that every element in $\mathbb{Z}$ that comes from $2\mathbb{Z}$ , i.e. the even integers, gets sent to the identity element or zero element $0$ of the abelian group $\mathbb{Z}/2\mathbb{Z}$ .

In the exact sequence

$0\rightarrow A\xrightarrow{f} B\xrightarrow{g} C\rightarrow 0$

The abelian group $B$ is sometimes referred to as the extension of the abelian group $C$ by the abelian group $A$ .

We recall the definition of the homology groups $H_{n}$ :

$H_{n}=\text{Ker }\partial_{n}/\text{Im }\partial_{n+1}$ .

We can see from this definition that a chain complex is an exact sequence (we can also say that the chain complex is acyclic) if all of its homology groups are zero. So in a way, the homology groups “measure” how much a chain complex “deviates” from being an exact sequence.

We also have the idea of a long exact complex, which usually comes from the homology groups of chain complexes which themselves form a short exact sequence. In order to discuss this we first need the notion of a chain map between chain complexes. If we have a chain complex

$...\xrightarrow{\partial_{A, n+3}}A_{n+2}\xrightarrow{\partial_{A, n+2}}A_{n+1}\xrightarrow{\partial_{A, n+1}}A_{n}\xrightarrow{\partial_{A, n}}A_{n-1}\xrightarrow{\partial_{A, n-1}}A_{n-2}\xrightarrow{\partial_{A, n-2}}...$

and another chain complex

$...\xrightarrow{\partial_{B, n+3}}B_{n+2}\xrightarrow{\partial_{B, n+2}}B_{n+1}\xrightarrow{\partial_{B, n+1}}B_{n}\xrightarrow{\partial_{B, n}}B_{n-1}\xrightarrow{\partial_{B, n-1}}B_{n-2}\xrightarrow{\partial_{B, n-2}}...$

a chain map is given by homomorphisms

$f_{n}: A_{n}\rightarrow B_{n}$ for all $n$

such that the homomorphisms $f_{n}$ commute with the boundary homomorphisms $\partial_{A, n}$ and $\partial_{B, n}$ , i.e.

$\partial_{B, n}\circ f_{n}=f_{n-1}\circ \partial_{A, n}$ for all $n$ .

A short exact sequence of chain complexes is then a short exact sequence

$0\rightarrow A_{n}\xrightarrow{f_{n}} B_{n}\xrightarrow{g_{n}} C_{n}\rightarrow 0$ for all $n$

where the homomorphisms $f_{n}$ and $g_{n}$ satisfy the conditions for them to form a chain map, i.e. they commute with the boundary homomorphisms in the sense shown above.

In the case that we have a short exact sequence of chain complexes, their homology groups will then form a long exact sequence:

$...\rightarrow H_{n}(A)\xrightarrow{f_{*}}H_{n}(B)\xrightarrow{g_{*}}H_{n}(C)\xrightarrow{\partial}H_{n-1}(A)\xrightarrow{f_{*}}...$

Long exact sequences are often used for calculating the homology groups of complicated topological spaces related in some way to simpler topological spaces whose homology groups are already known.

References:

Chain Complex on Wikipedia

Exact Sequence on Wikipedia

Algebraic Topology by Allen Hatcher

A Concise Course in Algebraic Topology by J. P. May

Abstract Algebra by David S. Dummit and Richard M. Foote

Eilenberg-MacLane Spaces, Spectra, and Generalized Cohomology Theories

December 20, 2016 / Anton Hilado / 2 Comments

In Homotopy Theory we explained the concept of homotopy and homotopy groups to study topological spaces and continuous functions from one topological space to another. Meanwhile, in Homology and Cohomology, we introduced the idea of homology and cohomology for the same purpose. In this post, we discuss one certain way in which homotopy and cohomology may be related to each other. Since homology and cohomology are related, being “duals” of each other in some sense, by extension this also relates homotopy and homology.

We recall first how we defined homology: Given a space $X$ , we construct a chain complex out of its subspaces. For subspaces of the same dimension, we must have the structure of an abelian group, so that these subspaces form chains on $X$ . The abelian group of $n$ -dimensional chains then have boundary functions or boundary maps (or boundary morphisms, since these chains form abelian groups) to the abelian group of $n-1$ -dimensional chains.

The homology groups $H_{n}(X)$ are then given as the quotient of the group of cycles (kernels of the $n$ -th boundary morphisms) by the group of boundaries (images of the $n+1$ -th boundary morphisms).

We also recall how we defined cohomology in terms of the homology. We define cochains on $X$ as the abelian group of the functions from chains on $X$ to some other abelian group $G$ . Together with the appropriate coboundary morphisms, they form what is called a cochain complex.

The cohomology groups $H^{n}(X,G)$ are defined, dually to the homology groups, as the quotient of the the group of cocycles (kernels of the $n$ -th coboundary morphisms) by the group of coboundaries (images of the $n-1$ -th coboundary morphisms).

We now review some important concepts in homotopy theory. The $n$ -th homotopy group $\pi_{n}(X)$ of a space $X$ is defined as the group $[S^{n},X]$ of homotopy classes of functions from the $n$ -dimensional sphere (also simply called the $n$ -sphere) to $X$ . equipped with appropriate basepoints. The homotopy group $\pi_{1}(X)$ is called the fundamental group of $X$ .

An Eilenberg-MacLane space, written $K(G,n)$ , for a certain group $G$ and a certain natural number $n$ , is a topological space characterized by the property that its $n$ -th homotopy group is $G$ and all its other homotopy groups are trivial. In Category Theory we mentioned that the fundamental group of a circle is the group of integers $\mathbb{Z}$ , corresponding to the number of times a loop winds around the circle before returning to its basepoint, with the direction taken into account. All the other homotopy groups of the circle are actually trivial, which makes it an Eilenberg-Maclane space $K(\mathbb{Z},1)$ .

For certain kinds of topological spaces called CW-complexes (spaces which can be built out of “cells” which are topological spaces homeomorphic to $n$ -dimensional open or closed balls) we have the following interesting relationship between homotopy and cohomology:

$\displaystyle \tilde{H}^{n}(X, G)\cong [X, K(G,n)]$

where the symbol “ $\cong$ ” denotes an isomorphism of groups and the $\tilde{H}^{n}(X,G)$ are the reduced cohomology groups, obtained by “dualizing” the augmented chain complex of reduced homology, which has

$\displaystyle ...\xrightarrow{\partial_{2}} C_{1}\xrightarrow{\partial_{1}} C_{0}\xrightarrow{\epsilon} \mathbb{Z}\rightarrow 0$

instead of the usual

$\displaystyle ...\xrightarrow{\partial_{2}} C_{1}\xrightarrow{\partial_{1}} C_{0}\xrightarrow{\partial_{0}} 0$

for the purpose of making the homology groups of a topological space $*$ consisting of a single point trivial. We have the following relation between the homology groups $H_{n}(X)$ and the reduced homology groups $\tilde{H}_{n}(X)$ :

$\displaystyle H_{n}(X)=\tilde{H}_{n}(X)\oplus H_{n}(*)$

Let $X_{+}$ be the topological space obtained by adjoining a disjoint basepoint to $X$ . Then

$\displaystyle H_{n}(X)=\tilde{H}_{n}(X_{+})$

Similarly, for cohomology, we have the following relations:

$\displaystyle H^{n}(X)=\tilde{H}^{n}(X)\oplus H^{n}(*)$

$\displaystyle H^{n}(X)=\tilde{H}^{n}(X_{+})$

The idea of using the homotopy classes of functions from one space to another to define some kind of “generalized cohomology theory” leads to the theory of “spectra” in algebraic topology (it should be noted that the word “spectra” or “spectrum” has many different meanings in mathematics, and in this post we are referring specifically to the concept in algebraic topology).

We first introduce some definitions. The wedge sum of two topological spaces $X$ and $Y$ with respective basepoints $x_{0}$ and $y_{0}$ , is the topological space, written $X\wedge Y$ , given by the quotient space $(X\coprod Y)/\sim$ under the identification $x_{0}\sim y_{0}$ . One can think of the space $X\wedge Y$ as being obtained from $X$ and $Y$ by gluing their basepoints together. For example, the wedge sum $S^{1}\wedge S^{1}$ of two circles can be thought of as the “figure eight”.

The smash product of two topological spaces $X$ and $Y$ , again with respective basepoints $x_{0}$ and $y_{0}$ , is the topological space, written $X\vee Y$ , given by the quotient space $(X\times Y)/(X\wedge Y)$ . What this means is that we take the cartesian product of $X$ and $Y$ , and then we collapse a copy of the wedge sum of $X$ and $Y$ containing the basepoint into the basepoint.

The suspension of a topological space $X$ is the topological space, written $SX$ , given by the quotient space $(X\times I)/\sim$ under the identifications $(x_{1},0)\sim (x_{2},0)$ and $(x_{1},1)\sim (x_{2},1)$ for any $x_{1}, x_{2}\in X$ , where $I$ is the unit interval $[0,1]$ . When $X$ is the circle $S^{1}$ , then $X\times I$ is the cylinder, and $SX$ is the cylinder with both ends collapsed into points. The space $SX$ also looks like two cones with their bases glued together.

The reduced suspension of a topological space $X$ with basepoint $x_{0}$ is the topological space, written $\Sigma X$ , given by the quotient space $(X\times I)/(X\times \{0\}\cup X\times \{1\}\cup\{x_{0}\}\times I)$ . This can be thought of as taking the suspension $SX$ of $X$ and then collapsing a copy of the unit interval containing the basepoint into the basepoint.

We note a couple of results regarding smash products and reduced suspensions. First, the smash product $S^{1}\vee X$ of the circle $S^{1}$ and a topological space $X$ is homeomorphic to the reduced suspension $\Sigma X$ of $X$ . Second, the smash product $S^{m}\vee S^{n}$ of an $m$ -dimensional sphere $S^{m}$ and an $n$ -dimensional sphere $S^{n}$ is homeomorphic to an $m+n$ -dimensional sphere $S^{m+n}$ .

The loop space $\Omega X$ of a space $X$ with a chosen basepoint $x_{0}$ is the topological space whose underlying set is the set of loops beginning and ending at $x_{0}$ and equipped with an appropriate topology called the compact-open topology.

We have the following relation between the concepts of reduced suspension and loop space:

$\displaystyle [\Sigma X, Y]\cong [X, \Omega Y]$

By the definition of homotopy groups and of Eilenberg-MacLane spaces, together with the properties of the reduced suspension and of the smash product, this implies that

$\Omega K(G,n)\cong K(G, n-1)$

where the symbol $\cong$ in this context denotes homeomorphism of topological spaces. We can repeat the process of taking the loop space to obtain

$\Omega^{2} K(G,n)=\Omega (\Omega K(G,n))\cong K(G, n-2)$

More generally, we will have

$\Omega^{m} K(G,n)=\Omega K(G,n)\cong K(G, n-m)$ for $m<n$

We now define the concepts of prespectra and spectra. A prespectrum $T$ is a sequence of spaces $T_{n}$ with basepoints and basepoint-preserving maps $\sigma: \Sigma T_{n}\rightarrow T_{n+1}$ . A spectrum $E$ is a prespectrum with adjoints $\tilde{\sigma}: E_{n}\rightarrow \Omega E_{n+1}$ which are homeomorphisms.

The Eilenberg-MacLane spaces form a spectrum, called the Eilenberg-MacLane spectrum. There are other spectra that will result in other generalized cohomology theories, which are defined to be functors (see Category Theory) from the category of pairs from pairs of topological spaces to the category of abelian groups, together with a natural transformation corresponding to a generalization of the boundary map, required to satisfy a set of conditions called the Eilenberg-Steenrod axioms. It follows from a certain theorem, called the Brown representability theorem, that every generalized cohomology theory comes from some spectrum, similar to how ordinary cohomology comes from the Eilenberg-MacLane spectrum.

References:

Eilenberg-MacLane Space on Wikipedia

Spectrum on Wikipedia

Eilenberg-Steenrod Axioms on Wikipedia

Algebraic Topology by Allen Hatcher

A Concise Course in Algebraic Topology by J. P. May

Theories and Theorems

Math and Physics for Everyone

algebraic topology

Prismatic Cohomology: An Overview

Some Useful Links: Knots in Physics and Number Theory

Covering Spaces

Some Useful Links on the Hodge Conjecture, Kahler Manifolds, and Complex Algebraic Geometry

An Intuitive Introduction to String Theory and (Homological) Mirror Symmetry

Differential Forms

Simplices

Vector Fields, Vector Bundles, and Fiber Bundles

Exact Sequences

Eilenberg-MacLane Spaces, Spectra, and Generalized Cohomology Theories